The Möbius Strip in Art and Design - A (select) Visual Survey

"I could be bounded in a nutshell and count myself a king of infinite space"

-William Shakespeare, Hamlet, 1603

Pick up a strip of paper, twist it one full turn, then tape the ends together. You've got a Möbius strip.

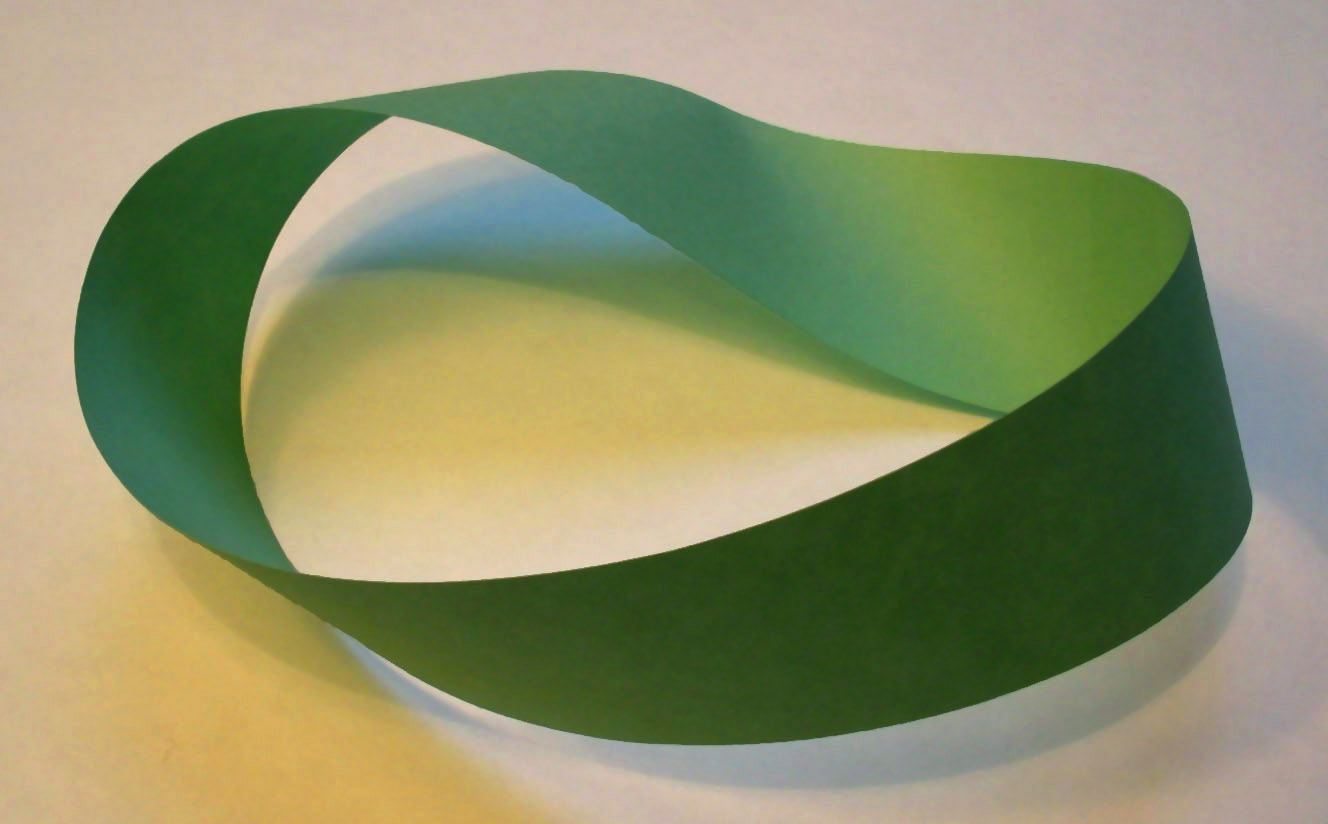
This seemigly simple object has a peculiar topological property - it has only one edge and only one side! Try coloring the edges in two different colors - it cant be done. A two-dimensional creature on the surface of the Möbius strip (say, for argument's sake, an ant with zero height) will perceive the surface as one infinitely long strip.

A Möbius Strip

The Möbius Strip has fascinated artists and mathematicians alike for a long time. What follows is a visual survey of the artwork this humble strip has inspired around the world.

Möbius Staircase - Nicky Stephens

Max Bill - Kontinuität outside Deutsche Bank's Headquarters in Frankfurt

At the Fermilab, Batavia, IL

Topological III - Robert Wilson, Harvard University, Cambridge, MA

Moebius Strip I (1961) - Maurits Cornelis Escher, wood engraving and woodcut in red, green, gold and black, printed from 4 blocks

Moebius Strip II (1963) - Maurits Cornelis Escher, woodcut in red, black and grey-green, printed from 3 blocks

LEGO Möbius Strip, Andrew Lipson

Möbius Gear, Concept by Tom Longtin

Aaron Hover's 3D printed model of Tom Longtin's concept

At this point I'm tempted to talk about Trefoil knots, Klein Bottles and higher dimensional non-orientable surfaces, but I will save them for another post!